trigonometric identities

hi guys,sohail here.I will be sharing some information regarding trigonometric identities.I hope you guys will find it helpful.Its quite similar  to the last blog because its a same topic but still information is important.



Trigonometric Identities
In mathematics, an "identity" is an equation which is always true. These can be "trivially" true, like "x =x" or usefully true, such as the Pythagorean Theorem's "a² + b² = c²" for right triangles. There are loads of trigonometric identities, but the following are the ones you're most likely to see and use.

Basic & Pythagorean, Angle-Sum & -Difference, Double-Angle, Half-Angle, Sum, Product

---

Basic and Pythagorean Identities

Notice how a "co-(something)" trig ratio is always the reciprocal of some "non-co" ratio. You can use this fact to help you keep straight that cosecant goes with sine and secant goes with cosine.

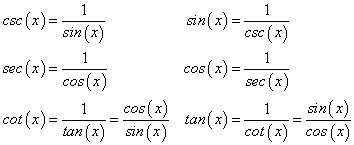
sin²(t) + cos²(t) = 1       tan²(t) + 1 = sec²(t)       1 + cot²(t) = csc²(t)

The above, because they involve squaring and the number 1, are the "Pythagorean" identities. You can see this clearly if you consider the unit circle, where sin(t) = y, cos(t) = x, and the hypotenuse is 1.

sin(–t) = –sin(t)       cos(–t) = cos(t)       tan(–t) = –tan(t)

Notice in particular that sine and tangent are odd functions, while cosine is an even function.
Angle-Sum and -Difference Identities

sin(α + β) = sin(α)cos(β) + cos(α)sin(β) 
sin(α – β) = sin(α)cos(β) – cos(α)sin(β) 
cos(α + β) = cos(α)cos(β) – sin(α)sin(β) 
cos(α – β) = cos(α)cos(β) + sin(α)sin(β)   
 

Double-Angle Identities

sin(2x) = 2sin(x)cos(x)

cos(2x) = cos²(x) – sin²(x) = 1 – 2sin²(x) = 2cos²(x) – 1

Half-Angle Identities   Copyright © Elizabeth Stapel 2010-2011 All Rights Reserved

The above identities can be re-stated as:

sin²(x) = ½[1 – cos(2x)]

cos²(x) = ½[1 + cos(2x)]

Proving an identity is very different in concept from solving an equation. Though you'll use many of the same techniques, they are not the same, and the differences are what can cause you problems.

An "identity" is a tautology, an equation or statement that is always true, no matter what. For instance,sin(x) = 1/csc(x) is an identity. To "prove" an identity, you have to use logical steps to show that one side of the equation can be transformed into the other side of the equation. You do not plug values into the identity to "prove" anything. There are infinitely-many values you can plug in. Are you really going to "prove" anything by listing three or four values where the two sides of the equation are equal? Of course not. And sometimes you'll be given an equation which is not an identity. If you plug a value in where the two sides happen to be equal, such as π/4 for the (false) identity sin(x) = cos(x), you could fool yourself into thinking that a mere equation is an identity. You'll have shot yourself in the foot. So let's don't do that.

To prove an identity, your instructor may have told you that you cannot work on both sides of the equation at the same time. This is correct. You can work on both sides together for a regular equation, because you're trying to find where the equation is true. When you are working with an identity, if you work on both sides and work down to where the sides are equal, you will only have shown that, if the starting equation is true, then you can arrive at another true equation. But you won't have proved, logically, that the original equation was actually true.

Since you'll be working with two sides of an equation, it might be helpful to introduce some notation, if you haven't seen it before. The "left-hand side" of an equation is denoted by LHS, and the "right-hand side" is denoted as RHS.

• Prove the identity 
It's usually a safe bet to start working on the side that appears to be more complicated. In this case, that would be the LHS. Another safe bet is to convert things to sines and cosines, and see where that leads. So my first step will be to convert the cotangent and cosecant into their alternative expressions:

Now I'll flip-n-multiply:

Now I can see that the sines cancel, leaving me with:

Then my proof of the identity is all of these steps, put together:

(I wrote them in the reverse order, to match the RHS.) The complete answer is all of the steps together, starting with the LHS and ending up with the RHS:

                                                                                                                                    

Solving Trigonometric Equations using Trigonometric Identities

Trigonometric identities are equations involving the trigonometric functions that are true for every value of the variables involved. You can use trigonometric identities along with algebraic methods to solve the trigonometric equations.

Extraneous Solutions

An extraneous solution is a root of a transformed equation that is not a root of the original equation because it was exclude from the domain of the original equation.
When you solve trigonometric equations, sometimes you can obtain an equation in one trigonometric function by squaring each side, but this technique may produce extraneous solutions.
Example :
Find all the solutions of the equation in the interval  .

The equation contains both sine and cosine functions.
We rewrite the equation so that it contains only cosine functions using the Pythagorean Identity sin² x = 1 – cos²x .

Factoring cos x we obtain, cos x (2 cos x + 1) = 0.
By using zero product property, we will get cos x = 0, and 2cos x+ 1 = 0 which yields cos x = –1/2.
In the interval [0, 2π), we know that cos x = 0 when x = π/2 andx = 3π/2. On the other hand, we also know that cos x = –1/2 when x = 2π/3 and x = 4π/3.
Therefore, the solutions of the given equation in the interval [0, 2π) are



                      
 Real world example: This provides an example of how trigonometric equations can be applied to real world scenarios.